Every ellipse can be changed back, through a translation or a rotation, to a standard ellipse whose centre is at the origin of the Cartesian plane and whose foci are on the x-axis or on the y‑axis.
Equations
The standard ellipse is the ellipse centered at the origin, whose vertices are \(S_1(a, 0)\) and \(S_2(−a, 0)\) on the x-axis and \(S_3(0, b)\) and \(S_4(0, −b)\) on the y-axis; its foci are the points with coordinates \(F_1(c, 0)\) and \(F_2(−c, 0)\).
If the ellipse is centred at the origin, the equations are the following:
- \(\dfrac{x^2}{a^2}\) + \(\dfrac{y^2}{b^2}\) = 1, where \({c^2}\) = \({a^2}\) – \({b^2}\) if the transverse axis is the x-axis:
The coordinates of the centre of the ellipse are: C(0, 0)
The coordinates of its foci are: F\(_{1}\)(c, 0) and F\(_{2}\)(−c, 0)
The coordinates of its vertices on the transverse axis are: S\(_{1}\)(a, 0) and S\(_{2}\)(−a, 0)
The coordinates of its vertices on the conjugate axis are: S\(_{3}\)(0, b) and S\(_{4}\)(0,−b)
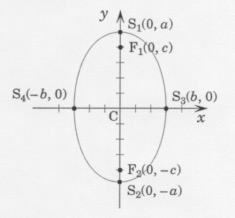
- \(\dfrac{y^2}{a^2}\) + \(\dfrac{x^2}{b^2}\) = 1, where \({c^2}\) = \({a^2}\) – \({b^2}\), if the transverse axis is the y-axis:
The coordinates of the centre of the ellipse are: C(0, 0)
The coordinates of its foci are: F\(_{1}\)(0, c) and F\(_{2}\)(0, −c)
The coordinates of its vertices on the transverse axis are: S\(_{1}\)(0, a) and S\(_{2}\)(0, −a)
The coordinates of its vertices on the conjugate axis are: S\(_{3}\)(b, 0) and S\(_{4}\)(−b, 0)
If the ellipse is not centred at the origin, the following data is obtained:
- \(\frac{(x-h)^{2}}{a^{2}}+\frac{(y-k)^{2}}{b^{2}}=1\), for an ellipse whose transverse axis is parallel to the x-axis:
The coordinates of the centre of the ellipse are: C(h,k).
The coordinates of its foci are: F\(_{1}\)(c + h, k) and F\(_{2}\)(−c + h, k).
The coordinates of its vertices on the transverse axis are: S\(_{1}\)(a + h, k) and S\(_{2}\)(−a + h, k).
The coordinates of its vertices on the conjugate axis are: S\(_{3}\)(h, b + k) and S\(_{4}\)(h,−b + k).
- \(\frac{(y-k)^{2}}{a^{2}}+\frac{(x-h)^{2}}{b^{2}}=1\), for an ellipse whose transverse axis is parallel to the y-axis:
The coordinates of its foci are: C(h, k)
The coordinates of its foci are: F\(_{1}\)(h, c + k) and F\(_{2}\)(h,−c + k)
The coordinates of its vertices on the transverse axis are: S\(_{1}\)(h, a + k) and S\(_{2}\)(h,−a + k)
The coordinates of its vertices on the conjugate axis are: S\(_{3}\)(b + h, k) and S\(_{4}\)(−b + h, k)
Example
In the illustration below, the standard ellipse was translated 6 units to the right and 3 units downward: (6, −3). Therefore, the following can be noted:
The coordinates of the centre of the ellipse are: C(6, −3)
The coordinates of the foci are: F\(_{1}\)(4 + 6, −3) and F\(_{2}\)(−4 + 6, −3)
The coordinates of its vertices on the transverse axis are: S\(_{1}\)(5 + 6, −3) and S\(_{2}\)(−5 + 6, −3)
The coordinates of its vertices on the conjugate axis are: S\(_{3}\)(6, 3 −3) and S\(_{4}\)(6, −3 + (−3))